本ページには広告が含まれています。
目次
16進数形式の文字列を単精度浮動小数点数に変換します。
- 構文
- Single = hexToSingle( hex )
- 引数
- hex 必須
- 16進数の値
- 戻り値
- 単精度浮動小数点数に変換した値
プログラム
//////////////////////////////////////////////////
// 【引数】
// hex : 16進数の値
// 【戻り値】
// 単精度浮動小数点数に変換した値
//////////////////////////////////////////////////
FUNCTION hexToSingle(hex)
IFB LENGTH(hex) <= 8 THEN
RESULT = VARTYPE(binToSingle(hexToBin(hex)), VAR_SINGLE)
ELSE
RESULT = ERR_VALUE
ENDIF
FEND
//////////////////////////////////////////////////
// 【引数】
// bin : 2進数
// signFlg : 符号付きならばTrue
// 【戻り値】
// 10進数に変換した値
//////////////////////////////////////////////////
FUNCTION binToDec(bin, signFlg = TRUE)
DIM dec = 0
DIM decimalFlg = IIF(POS(".", bin), TRUE, FALSE)
IFB COPY(bin, 1, 1) = "1" AND signFlg THEN
DIM msb = IIF(decimalFlg, POS(".", bin) - 1, LENGTH(bin))
DIM lsb = IIF(decimalFlg, POS(".", bin) - LENGTH(bin), 0)
DIM dec2 = POWER(2, msb) - 1
FOR i = -1 TO lsb STEP -1
dec2 = dec2 + POWER(2, i)
NEXT
DIM a = binToDec(bin, FALSE)
DIM b = dec2
dec = -1 * (bitXor(a, b) + POWER(2, lsb))
ELSE
IFB decimalFlg THEN
DIM integer = COPY(bin, 1, POS(".", bin) - 1)
DIM decimal = COPY(bin, POS(".", bin) + 1)
FOR i = 1 TO LENGTH(decimal)
dec = dec + COPY(decimal, i, 1) * POWER(2, -1 * i)
NEXT
ELSE
integer = bin
ENDIF
FOR i = 1 TO LENGTH(integer)
dec = dec + COPY(integer, i, 1) * POWER(2, LENGTH(integer) - i)
NEXT
ENDIF
RESULT = dec
FEND
//////////////////////////////////////////////////
// 【引数】
// bin : 2進数の値
// 【戻り値】
// 単精度浮動小数点数に変換した値
//////////////////////////////////////////////////
FUNCTION binToSingle(bin)
IFB LENGTH(bin) = 32 THEN
DIM s = COPY(bin, 1, 1)
s = POWER(-1, VAL(s))
DIM e = COPY(bin, 2, 8)
e = POWER(2, binToDec(e, FALSE) - 127)
DIM m = COPY(bin, 10)
m = binToDec("1." + m, FALSE)
RESULT = VARTYPE(s * e * m, VAR_SINGLE)
ELSE
RESULT = ERR_VALUE
ENDIF
FEND
//////////////////////////////////////////////////
// 【引数】
// num : 10進数もしくは2進数の値
// bit : ビット
// 【戻り値】
// ビットを反転した値
//////////////////////////////////////////////////
FUNCTION bitNot(num, bit = EMPTY)
IFB isString(num) THEN
DIM res = ""
FOR i = 1 TO LENGTH(num)
DIM str = COPY(num, i, 1)
IFB str = "0" OR str = "1" THEN
res = res + (1 - VAL(str))
ELSE
res = res + str
ENDIF
NEXT
RESULT = res
ELSE
DIM exponent = IIF(bit = EMPTY, CEIL(LOGN(2, num + 1)), bit)
RESULT = POWER(2, exponent) - num - 1
ENDIF
FEND
//////////////////////////////////////////////////
// 【引数】
// arg1 : 数値1(10進数)
// arg2 : 数値2(10進数)
// 【戻り値】
// 2つの数値のビット毎の排他的論理和
//////////////////////////////////////////////////
FUNCTION bitXor(arg1, arg2)
DIM args[1] = arg1, arg2
DIM bins[1]
DIM decimals[1]
DIM integers[1]
DIM keta[1]
IFB ABS(arg1) <> arg1 OR ABS(arg2) <> arg2 THEN
RESULT = ERR_VALUE
EXIT
ENDIF
FOR i = 0 TO 1
bins[i] = decToBin(args[i])
decimals[i] = 0
IFB POS(".", bins[i]) <> 0 THEN
integers[i] = COPY(bins[i], 1, POS(".", bins[i]) - 1)
decimals[i] = COPY(bins[i], POS(".", bins[i]) + 1)
ELSE
integers[i] = bins[i]
ENDIF
NEXT
keta[0] = IIF(LENGTH(integers[0]) > LENGTH(integers[1]), LENGTH(integers[0]), LENGTH(integers[1]))
integers[0] = strPad(integers[0], keta[0], "0", LEFT)
integers[1] = strPad(integers[1], keta[0], "0", LEFT)
keta[1] = IIF(LENGTH(decimals[0]) > LENGTH(decimals[1]), LENGTH(decimals[0]), LENGTH(decimals[1]))
decimals[0] = strPad(decimals[0], keta[1], "0", RIGHT)
decimals[1] = strPad(decimals[1], keta[1], "0", RIGHT)
DIM bin = ""
FOR i = 1 TO keta[0]
bin = bin + (VAL(COPY(integers[0], i, 1)) XOR VAL(COPY(integers[1], i, 1)))
NEXT
bin = bin + "."
FOR i = 1 TO keta[1]
bin = bin + (VAL(COPY(decimals[0], i, 1)) XOR VAL(COPY(decimals[1], i, 1)))
NEXT
RESULT = binToDec(bin)
FEND
//////////////////////////////////////////////////
// 【引数】
// dec : 10進数
// signFlg : 符号付きならばTrueを指定
// digits : 変換した2進数の桁数合わせを自動で行うかを示すブール値、もしくは桁数を表す数値(8,16,24,32,64のいずれか)を指定
// errorMsg : エラーメッセージを出力するかを示すブール値
// 【戻り値】
// 2進数に変換した値
//////////////////////////////////////////////////
FUNCTION decToBin(dec, signFlg = FALSE, digits = FALSE, errorMsg = FALSE)
// 負数で符号なしならばエラー値を返す
IFB dec < 0 AND signFlg = FALSE THEN
PRINT "負数の場合signFlgにTrueを指定してください"
RESULT = ERR_VALUE
EXIT
ENDIF
// digitsのビット数が足りなければエラー値を返す、負数なら1桁多く取る
IFB VARTYPE(digits) <> VAR_BOOLEAN AND digits < CEIL(LOGN(2, ABS(dec))) + IIF(dec < 0, 1, 0) THEN
PRINT "ビット数が足りません"
RESULT = ERR_VALUE
EXIT
ENDIF
// signFlgがTrueかつdigitsがFalseならばエラー値を返す
IFB signFlg AND !digits THEN
PRINT "signFlgがTrueのときdigitsはFalse以外を選択してください"
RESULT = ERR_VALUE
EXIT
ENDIF
// bin:2進数に変換した結果を代入する変数
DIM bin = ""
DIM msg = ""
DIM isError = FALSE
DIM decimalFlg = IIF(POS(".", dec) <> 0, TRUE, FALSE)
DIM negativeFlg = IIF(dec < 0, TRUE, FALSE)
dec = ABS(dec)
// (1) 10進数を整数部と小数部に分ける
DIM integer = IIF(decimalFlg, COPY(dec, 1, POS(".", dec) - 1), dec)
DIM decimal = IIF(decimalFlg, "0." + COPY(dec, POS(".", dec) + 1), 0)
// (2) 10進数(整数部)を2進数に変換する。
REPEAT
bin = (integer MOD 2) + bin
integer = INT(integer / 2)
UNTIL integer = 0
// (3) 10進数(小数部)を2進数に変換する。
IFB decimalFlg THEN
bin = bin + "."
DIM loop = 0
REPEAT
loop = loop + 1
decimal = decimal * 2
bin = bin + IIF(decimal >= 1, "1", "0")
IF decimal > 1 THEN decimal = decimal - 1
UNTIL decimal = 1 OR loop > 64
ENDIF
// digitsがFALSE以外なら
IFB digits THEN
// (4) 2進数の桁合わせを行う
DIM tmp = bin
DIM binInteger = TOKEN(".", tmp)
DIM binDecimal = TOKEN(".", tmp)
// 整数部、小数部を4bit単位になるまで拡張
// 整数部、4の倍数になるまで整数部の先頭に'0'を追加
IF LENGTH(binInteger) MOD 4 <> 0 THEN binInteger = strRepeat("0", 4 - LENGTH(binInteger) MOD 4) + binInteger
// 小数部、4の倍数になるまで小数部の末尾に'0'を追加
IF LENGTH(binDecimal) MOD 4 <> 0 THEN binDecimal = binDecimal + strRepeat("0", 4 - LENGTH(binDecimal) MOD 4)
DIM digit = LENGTH(binInteger + binDecimal)
// 10進数の場合、一旦自動調整を行う
integer = INT(dec)
IF signFlg AND COPY(binInteger, 1, 1) = "1" THEN binInteger = strRepeat("0", 4) + binInteger
IFB signFlg THEN
IFB integer >= -128 AND integer <= 127 THEN // -2^7〜2^7-1
binInteger = strRepeat("0", 8 - LENGTH(binInteger)) + binInteger
ELSEIF integer >= -32768 AND integer <= 32767 THEN // -2^15〜2^15-1
binInteger = strRepeat("0", 16 - LENGTH(binInteger)) + binInteger
ELSEIF integer >= -8388608 AND integer <= 8388607 THEN // -2^23〜2^23-1
binInteger = strRepeat("0", 24 - LENGTH(binInteger)) + binInteger
ELSEIF integer >= -2147783648 AND integer <= 2147483647 THEN // -2^31〜2^31-1
binInteger = strRepeat("0", 32 - LENGTH(binInteger)) + binInteger
ELSE
binInteger = strRepeat("0", 64 - LENGTH(binInteger)) + binInteger
ENDIF
ELSE
IFB integer <= 255 THEN // 2^8-1
binInteger = strRepeat("0", 8 - LENGTH(binInteger)) + binInteger
ELSEIF integer <= 65535 THEN // 2^16-1
binInteger = strRepeat("0", 16 - LENGTH(binInteger)) + binInteger
ELSEIF integer <= 16777215 THEN // 2^24-1
binInteger = strRepeat("0", 24 - LENGTH(binInteger)) + binInteger
ELSEIF integer <= 4294967295 THEN // 2^32-1
binInteger = strRepeat("0", 32 - LENGTH(binInteger)) + binInteger
ELSE
binInteger = strRepeat("0", 64 - LENGTH(binInteger)) + binInteger
ENDIF
ENDIF
totalDigits = LENGTH(binInteger + binDecimal)
IFB totalDigits > 64 THEN
DIM del32 = totalDigits - 32
DIM del64 = totalDigits - 64
IFB del32 = LENGTH(binDecimal) AND digits <> 64 THEN
binDecimal = ""
msg = "32bitを超えたため、小数点以下を削除しました"
ELSEIF del32 < LENGTH(binDecimal) AND digits <> 64 THEN
binDecimal = COPY(binDecimal, 1, LENGTH(binDecimal) - del32)
msg = "32bitを超えたため、小数点以下の一部を削除しました"
ELSEIF del64 = LENGTH(binDecimal) AND del64 <> 0 THEN
binDecimal = ""
msg = "64bitを超えたため、小数点以下を削除しました"
ELSEIF del64 < LENGTH(binDecimal) THEN
binDecimal = COPY(binDecimal, 1, LENGTH(binDecimal) - del64)
msg = "64bitを超えたため、小数点以下の一部を削除しました"
ELSE
msg = "64bitを超えるため、変換できません"
isError = TRUE
ENDIF
ENDIF
// 整数部、小数部の合計桁数を8,16,24,32,64bit単位になるまで拡張
digit = LENGTH(binInteger + binDecimal)
DIM array[] = 8, 16, 24, 32, 64
FOR item IN array
IFB digit <= item THEN
binInteger = strRepeat("0", item - digit) + binInteger
BREAK
ENDIF
NEXT
// 指定ビットに調整
// 合計桁数の再設定
totalDigits = LENGTH(binInteger + binDecimal)
IFB digits = TRUE THEN
// 桁合わせを自動調整
IFB totalDigits > 64 THEN
len = LENGTH(binInteger + binDecimal)
WHILE LENGTH(binInteger) > 8 AND len > digits
IFB COPY(binInteger, 1, 4) = "0000" THEN
binInteger = COPY(binInteger, 5)
len = len - 4
ELSE
BREAK
ENDIF
WEND
WHILE LENGTH(binDecimal) > 4 AND LENGTH(binInteger + binDecimal) > digits
IFB COPY(binDecimal, LENGTH(binDecimal) - 4) = "0000" THEN
binDecimal = COPY(binDecimal, 1, LENGTH(binDecimal) - 4)
ELSE
BREAK
ENDIF
WEND
tmp = binInteger + "." + binDecimal
binInteger = COPY(tmp, 1, POS(".", tmp) - 1)
binDecimal = COPY(tmp, POS(".", tmp) + 1)
totalDigits = LENGTH(binInteger + binDecimal)
IFB totalDigits > 64 THEN
isError = TRUE
msg = "64bitを超えたため変換できません"
ENDIF
ENDIF
ELSE
// 指定ビットに調整
IFB totalDigits <= digits THEN
binInteger = strPad(binInteger, digits - LENGTH(binDecimal), "0", LEFT)
ELSE
// 桁あふれ調整
totalDigits = LENGTH(binInteger + binDecimal)
len = LENGTH(binInteger + binDecimal)
WHILE LENGTH(binInteger) > 8 AND len > digits
IFB COPY(binInteger, 1, 4) = "0000" THEN
binInteger = COPY(binInteger, 5)
len = len - 4
ELSE
BREAK
ENDIF
WEND
WHILE LENGTH(binDecimal) > 4 AND LENGTH(binInteger + binDecimal) > digits
IFB COPY(binDecimal, LENGTH(binDecimal) - 4) = "0000" THEN
binDecimal = COPY(binDecimal, 1, LENGTH(binDecimal) - 4)
ELSE
BREAK
ENDIF
WEND
tmp = binInteger + "." + binDecimal
binInteger = COPY(tmp, 1, POS(".", tmp) - 1)
binDecimal = COPY(tmp, POS(".", tmp) + 1)
len = LENGTH(binInteger + binDecimal)
IFB len > digits THEN
DIM deleteLength = len - digits
IFB deleteLength = LENGTH(binDecimal) THEN
binDecimal = ""
msg = "指定ビット数にするため小数点以下を削除しました"
ELSEIF deleteLength < LENGTH(binDecimal) THEN
binDecimal = COPY(binDecimal, 1, LENGTH(binDecimal) - deleteLength)
msg = "指定ビット数にするため小数点以下の一部を削除しました"
ELSE
isError = TRUE
msg = "指定ビット数では変換できません"
ENDIF
ENDIF
ENDIF
ENDIF
bin = binInteger + IIF(binDecimal <> "", "." + binDecimal, "")
// (5) 入力値がマイナスのため、2進数をマイナス値に変換する
IFB negativeFlg THEN
// 1の補数
bin = bitNot(bin)
// 2の補数
DIM res = ""
DIM carry = "1"
FOR i = LENGTH(bin) TO 1 STEP -1
IFB carry = "1" THEN
SELECT COPY(bin, i, 1)
CASE "0"
res = "1" + res
carry = 0
CASE "1"
res = "0" + res
DEFAULT
res = COPY(bin, i, 1) + res
SELEND
ELSE
res = COPY(bin, i, 1) + res
ENDIF
NEXT
bin = res
ENDIF
ENDIF
IF errorMsg AND msg <> "" THEN PRINT msg
RESULT = IIF(isError, ERR_VALUE, bin)
FEND
//////////////////////////////////////////////////
// 【引数】
// hex : 16進数
// 【戻り値】
// 2進数に変換した値
//////////////////////////////////////////////////
FUNCTION hexToBin(hex)
HASHTBL hb
hb["0"] = "0000"; hb["1"] = "0001"; hb["2"] = "0010"; hb["3"] = "0011";
hb["4"] = "0100"; hb["5"] = "0101"; hb["6"] = "0110"; hb["7"] = "0111";
hb["8"] = "1000"; hb["9"] = "1001"; hb["A"] = "1010"; hb["B"] = "1011";
hb["C"] = "1100"; hb["D"] = "1101"; hb["E"] = "1110"; hb["F"] = "1111";
DIM bin = ""
IFB POS(".", hex) <> 0 THEN
FOR i = 1 TO LENGTH(hex)
DIM str = COPY(hex, i, 1)
IF str = "." THEN bin = bin + "."
bin = bin + hb[str]
NEXT
ELSE
FOR i = 1 TO LENGTH(hex)
bin = bin + hb[COPY(hex, i, 1)]
NEXT
ENDIF
RESULT = bin
FEND
//////////////////////////////////////////////////
// 【引数】
// expr : 評価する式
// truepart : 評価した式がTrueのときに返す値
// falsepart : 評価した式がFalseのときに返す値
// 【戻り値】
// truepart : 評価した式がTrueのとき、falsepart : 評価した式がFalseのとき
//////////////////////////////////////////////////
FUNCTION IIF(expr, truepart, falsepart)
IFB EVAL(expr) THEN
RESULT = truepart
ELSE
RESULT = falsepart
ENDIF
FEND
//////////////////////////////////////////////////
// 【引数】
// variable : 型を調べる変数
// 【戻り値】
// : TRUE : 与えられた変数が文字列型である、
// FALSE : 与えられた変数が文字列型でない、 :
//////////////////////////////////////////////////
FUNCTION isString(variable)
RESULT = IIF(VARTYPE(variable) = VAR_ASTR OR VARTYPE(variable) = VAR_USTR, TRUE, FALSE)
FEND
//////////////////////////////////////////////////
// 【引数】
// num : 符号を求める数値
// 【戻り値】
// 1 : 正の数、0 : ゼロ、-1 : 負の数、ERR_VALUE : それ以外
//////////////////////////////////////////////////
FUNCTION sign(num)
SELECT TRUE
CASE !CHKNUM(num)
RESULT = ERR_VALUE
CASE num > 0
RESULT = 1
CASE num = 0
RESULT = 0
CASE num < 0
RESULT = -1
SELEND
FEND
//////////////////////////////////////////////////
// 【引数】
// input : 入力文字列
// length : 埋めたあとの長さ
// str : 埋める文字
// type : 埋める方向
// 【戻り値】
// 指定文字で埋めた文字列
//////////////////////////////////////////////////
FUNCTION strPad(input, length, str = " ", type = RIGHT)
DIM s = ""
SELECT type
CASE LEFT
FOR i = 1 TO CEIL((length - LENGTH(input)) / LENGTH(str))
s = s + str
NEXT
input = COPY(s, 1, length - LENGTH(input)) + input
CASE RIGHT
FOR i = 1 TO CEIL((length - LENGTH(input)) / LENGTH(str))
s = s + str
NEXT
input = input + COPY(s, 1, length - LENGTH(input))
SELEND
RESULT = input
FEND
//////////////////////////////////////////////////
// 【引数】
// inputs : 繰り返す文字列
// multiplier : inputsを繰り返す回数
// 【戻り値】
// inputsをmultiplier回を繰り返した文字列を返します
//////////////////////////////////////////////////
FUNCTION strRepeat(inputs, multiplier)
DIM res = ""
FOR n = 1 TO multiplier
res = res + inputs
NEXT
RESULT = res
FEND単精度浮動小数点数/Single
- 正の値
- \({1.1754944 \times 10^{-38}}_{(10)}\) ~ \({3.40282339717 \times 10^{38}}_{(10)}\)
- 負の値
- \({-3.40282339717 \times 10^{38}}_{(10)}\) ~ \({-1.1754944 \times 10^{-38}}_{(10)}\)
 \[(-1)^{\mathrm{sign}} \times 2^{\mathrm{exponent} - \mathrm{exponent}\ \ \mathrm{bias}} \times 1.\mathrm{mantissa}\]
\[(-1)^{\mathrm{sign}} \times 2^{\mathrm{exponent} - \mathrm{exponent}\ \ \mathrm{bias}} \times 1.\mathrm{mantissa}\]
- \(\mathrm{sign}:符号\)
- \(\mathrm{exponent}:指数部\)
- \(\mathrm{exponent} \mathrm{bias}:指数部バイアス\)
- \(\mathrm{mantissa}:仮数部\)
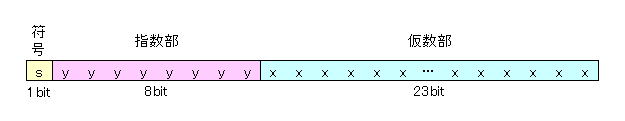
- \(s:符号部\)
- \(xxx:仮数部\)
- \(yyy:指数部\)
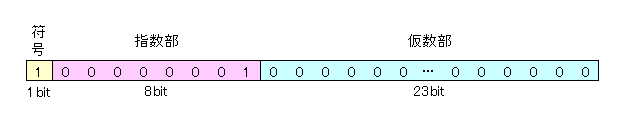
- 符号ビット
- 1ビット
- 指数部の幅
- 8ビット(ゲタ履き表現)
- 仮数部の幅
- 23ビット
- 有効桁数
- \(\log_{10} 2^{23} = 23\log_{10} 2 = 23 * 0.301029957 = 6.923\)
符号ビットは表現する数値の符号(正負)を示します。指数部は8ビットで、符号なし整数として見た場合は0~255の値をとり、0のときは0か非正規化数であることを、1~254のときは-127のゲタ履き(バイアス付き)表現で-126~127の指数を、255のときは無限大またはNaNであることを示します。
指数部が0(0および非正規化数)の場合を除き、仮数部で表現されるビットパターンのさらにひとつ上の桁に暗黙の1のビットがあるとみなす表現法(ケチ表現)により、正規化された数の精度は、24ビットである。十進に換算したときに表現できる桁数は\(\log_{10} 2^{24} \approx 7.225\)桁となります。
指数部の符号化方式
単精度浮動小数点数の指数部はオフセット(ゲタ履き)表現を使って符号化されており、指数値が0のときオフセット値(バイアス値)は127である。
- \(E_{min}=01_{H}-7F_{H}=-126\)
- \(E_{max}=FE_{H}-7F_{H}=127\)
- \(exponent\ \ bias(指数バイアス)={7F}_{H}=127\)
\(00_{H}\)と\(FF_{H}\)は予約された指数値です。
正の最小値(単精度)
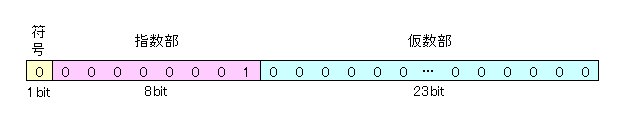
- 符号
- \((-1)^{0}\)
- 仮数部
- \(xxx = 000~0000~0000~0000~0000~0000\)
\(1.xxx=1.00000000000000000000000_{(2)}={2^{0}}_{(10)}=1.0_{(10)}\) - 指数部
- \(yyy = 0000~0001_{(2)}=1_{(10)}\)
\({2^{1-127}}_{(10)}={2^{-126}}_{(10)}\)=\({\frac{1}{2^{126}}}_{(10)}\)=\({1.1754944\times10^{-38}}_{(10)}\) - 符号×仮数部×指数部
- \[ \begin{eqnarray} value_{min}&=&(-1)^{0} \times 1 \times \frac{1}{2^{126}}\\ &=&1.175494351 \times 10^{-38} \end{eqnarray} \]
正の最大値(単精度)
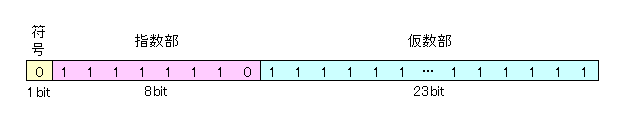
- 符号
- \((-1)^{0}\)
- 仮数部
- \(xxx = 111~1111~1111~1111~1111~1111_{(2)}\)
\(1.xxx=1.11111111111111111111111\)
\[2^{0},2^{-1},2^{-2},…,2^{-23}\]- \(初項:a=1\)
- \(公比:r=0.5\)
- \(項数:n=24\)
- 指数部
- \(yyy = 1111~1110_{(2)}=254\)
\({2^{254-127}}_{(10)} = {2^{127}}_{(10)}={1.7014118\times10^{38}}_{(10)}\) - 符号×仮数部×指数部
- \[ \begin{eqnarray} value_{max}&=&(-1)^{0} \times \frac{1-0.5^{24}}{0.5} \times 2^{127} \\ &=&3.4028235 \times 10^{38} \end{eqnarray} \]
負の最小値(単精度)
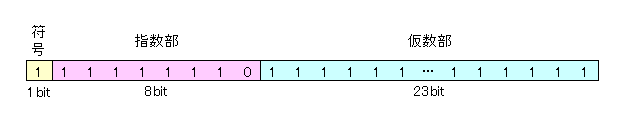
- 符号
- \((-1)^{1}\)
- 仮数部
- \(xxx = 111~1111~1111~1111~1111~1111_{(2)}\)
\(1.xxx=1.11111111111111111111111_{(2)}\)
\(S=\frac{a(a-r^{n})}{1-r}=\frac{1-0.5^{24}}{1-0.5}=\frac{1-0.5^{24}}{0.5}=1.99999988079_{(10)}\) - 指数部
- \(yyy = 1111~1110_{(2)}=254\)
\({2^{254-127}}_{(10)}={2^{127}}_{(10)}=1.7014118 \times {10^{38}}_{(10)}\) - 符号×仮数部×指数部
- \[ \begin{eqnarray} value_{min}&=&(-1)^{1} \times \frac{1-0.5^{24}}{0.5} \times 2^{127}\\ &=&-3.4028235 \times 10^{38} \end{eqnarray} \]
負の最大値(単精度)
- 符号
- \((-1)^{1}\)
- 仮数部
- \(xxx = 000~0000~0000~0000~0000~0000_{(2)}\)
\(1.xxx=1.00000000000000000000000_{(2)}\)
\({2^{0}}_{(10)}=1.0_{(10)}\) - 指数部
- \(yyy = 0000~0001_{(2)}\)
\({2^{1-127}}_{(10)}={2^{-126}}_{(10)}\)=\({\frac{1}{2^{126}}}_{(10)}\)=\({1.1754944\times10^{-38}}_{(10)}\) - 符号×仮数部×指数部
- \[ \begin{eqnarray} value_{max}&=&(-1)^{1} \times 1.0 \times \frac{1}{2^{126}} \\ &=&-1.175494351 \times 10^{-38} \end{eqnarray} \]
使い方
引数に指定された16進数表記の文字列を単精度浮動小数点数に変換します。
PRINT hexToSingle("3F200000")
PRINT hexToSingle("C1810000")- 結果
0.625 -16.125

